A星算法Java实现
在一张地图中,绘制从起点移动到终点的最优路径,地图中会有障碍物,必须绕开障碍物。
(如果有路径)采用“结点与结点的父节点”的关系从最终结点回溯到起点,得到路径。
A星算法的代价计算使用了被称作是启发式的代价函数。 先说明一下各符号意义:G表示的是从起点到当前结点的实际路径代价(为啥叫实际?就是已经走过了,边走边将代价计算好了);H表示当前结点到达最终结点的估计代价(为啥叫估计?就是还没走过,不知道前面有没障碍、路通不通,所以只能用估计);F表示当前结点所在路径从起点到最终点预估的总路径代价。
G的计算方式:计算方式有挺多种的,这里我们就用这种吧,假设每个结点代表一个正方形,横竖移动距离:斜移动距离=1:1.4(根号2),我们取个整数10和14吧,也就是说当前结点G值=父节点的G+(10或14)。
H的计算方式:估价计算也有很多种方式,我们这里使用“曼哈顿”法,H=(|当前结点x值-最终结点x值|+|当前结点y值-最终结点y值|) * 横竖移动代价值("||"表示绝对值)。
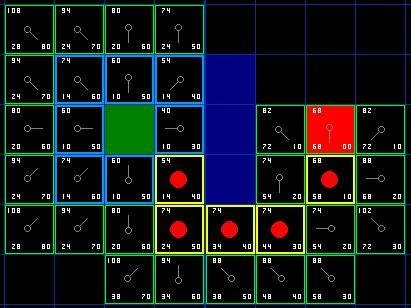
如下图(图不是自己做的,从网上借来的,自己画的话~...惨不忍睹!)
在A星算法中,需要使用两个辅助表来记录结点。 一个用于记录可被访问的结点,成为Open表;一个是记录已访问过的结点,称为Close表。 这两个表决定了算法的结束:条件是最终结点在Close表中(找到路径)或Open表为空(找不到了路径)。
上面的理解的话,现在就来移动当前的节点,寻找路径。
每次从Open表中取出F值最小的结点出来(这里我们使用优先队列来处理比较好),作为当前结点;然后将当前结点的所有邻结点按照邻结点规则加入到Open表中;最后将当前结点放入Close表中,这里就是每次循环的执行内容。
邻结点规则: (1) 当邻结点不在地图中,不加入Open表; (2) 当邻结点是障碍物,不加入Open表; (3) 当邻结点在Close表中,不加入Open表; (4) 当邻结点不在Open中,加入Open表,设该邻结点的父节点为当前结点; (5) **当邻结点在Open表中,我们需要做个比较:如果邻结点的G值>当前结点的G值+当前结点到这个邻结点的代价,那么修改该邻结点的父节点为当前的结点(因为在Open表中的结点除了起点,都会有父节点),修改G值=当前结点的G值+当前结点到这个邻结点的代价 **
蓝色框框表示在Close表中,绿色的框框表示在Open表中
最后回溯得到路径
(1) 代表地图二值二维数组(0表示可通路,1表示路障)
int[][] maps = {
{ 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0 },
{ 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0 },
{ 0, 0, 1, 1, 0, 0, 0, 0, 0, 0, 1, 1, 1, 0, 0 },
{ 0, 0, 0, 1, 0, 0, 0, 0, 0, 1, 1, 0, 0, 0, 0 },
{ 0, 0, 0, 1, 0, 0, 0, 0, 1, 1, 0, 0, 0, 0, 0 },
{ 0, 0, 0, 1, 0, 0, 0, 0, 1, 0, 0, 0, 0, 0, 0 },
{ 0, 0, 0, 1, 0, 0, 0, 0, 1, 0, 0, 0, 0, 0, 0 }
};(2) 按照二维数组的特点,坐标原点在左上角,所以y是高,x是宽,y向下递增,x向右递增,我们将x和y封装成一个类,好传参,重写equals方法比较坐标(x,y)是不是同一个。
public class Coord
{
public int x;
public int y;
public Coord(int x, int y)
{
this.x = x;
this.y = y;
}
@Override
public boolean equals(Object obj)
{
if (obj == null) return false;
if (obj instanceof Coord)
{
Coord c = (Coord) obj;
return x == c.x && y == c.y;
}
return false;
}
}(3) 封装路径结点类,字段包括:坐标、G值、F值、父结点,实现Comparable接口,方便优先队列排序。
public class Node implements Comparable<Node>
{
public Coord coord; // 坐标
public Node parent; // 父结点
public int G; // G:是个准确的值,是起点到当前结点的代价
public int H; // H:是个估值,当前结点到目的结点的估计代价
public Node(int x, int y)
{
this.coord = new Coord(x, y);
}
public Node(Coord coord, Node parent, int g, int h)
{
this.coord = coord;
this.parent = parent;
G = g;
H = h;
}
@Override
public int compareTo(Node o)
{
if (o == null) return -1;
if (G + H > o.G + o.H)
return 1;
else if (G + H < o.G + o.H) return -1;
return 0;
}
}(4) 最后一个数据结构是A星算法输入的所有数据,封装在一起,传参方便。:grin:
public class MapInfo
{
public int[][] maps; // 二维数组的地图
public int width; // 地图的宽
public int hight; // 地图的高
public Node start; // 起始结点
public Node end; // 最终结点
public MapInfo(int[][] maps, int width, int hight, Node start, Node end)
{
this.maps = maps;
this.width = width;
this.hight = hight;
this.start = start;
this.end = end;
}
}(1) 在算法里需要定义几个常量来确定:二维数组中哪个值表示障碍物、二维数组中绘制路径的代表值、计算G值需要的横纵移动代价和斜移动代价。
public final static int BAR = 1; // 障碍值
public final static int PATH = 2; // 路径
public final static int DIRECT_VALUE = 10; // 横竖移动代价
public final static int OBLIQUE_VALUE = 14; // 斜移动代价(2) 定义两个辅助表:Open表和Close表。Open表的使用是需要取最小值,在这里我们使用Java工具包中的优先队列PriorityQueue,Close只是用来保存结点,没其他特殊用途,就用ArrayList。
Queue<Node> openList = new PriorityQueue<Node>(); // 优先队列(升序)
List<Node> closeList = new ArrayList<Node>();(3) 定义几个布尔判断方法:最终结点的判断、结点能否加入open表的判断、结点是否在Close表中的判断。
/**
* 判断结点是否是最终结点
*/
private boolean isEndNode(Coord end,Coord coord)
{
return coord != null && end.equals(coord);
}
/**
* 判断结点能否放入Open列表
*/
private boolean canAddNodeToOpen(MapInfo mapInfo,int x, int y)
{
// 是否在地图中
if (x < 0 || x >= mapInfo.width || y < 0 || y >= mapInfo.hight) return false;
// 判断是否是不可通过的结点
if (mapInfo.maps[y][x] == BAR) return false;
// 判断结点是否存在close表
if (isCoordInClose(x, y)) return false;
return true;
}
/**
* 判断坐标是否在close表中
*/
private boolean isCoordInClose(Coord coord)
{
return coord!=null&&isCoordInClose(coord.x, coord.y);
}
/**
* 判断坐标是否在close表中
*/
private boolean isCoordInClose(int x, int y)
{
if (closeList.isEmpty()) return false;
for (Node node : closeList)
{
if (node.coord.x == x && node.coord.y == y)
{
return true;
}
}
return false;
}(4) 计算H值,“曼哈顿” 法,坐标分别取差值相加
private int calcH(Coord end,Coord coord)
{
return (Math.abs(end.x - coord.x) + Math.abs(end.y - coord.y)) * DIRECT_VALUE;
}(5) 从Open列表中查找结点
private Node findNodeInOpen(Coord coord)
{
if (coord == null || openList.isEmpty()) return null;
for (Node node : openList)
{
if (node.coord.equals(coord))
{
return node;
}
}
return null;
}(6) 添加邻结点到Open表
/**
* 添加所有邻结点到open表
*/
private void addNeighborNodeInOpen(MapInfo mapInfo,Node current)
{
int x = current.coord.x;
int y = current.coord.y;
// 左
addNeighborNodeInOpen(mapInfo,current, x - 1, y, DIRECT_VALUE);
// 上
addNeighborNodeInOpen(mapInfo,current, x, y - 1, DIRECT_VALUE);
// 右
addNeighborNodeInOpen(mapInfo,current, x + 1, y, DIRECT_VALUE);
// 下
addNeighborNodeInOpen(mapInfo,current, x, y + 1, DIRECT_VALUE);
// 左上
addNeighborNodeInOpen(mapInfo,current, x - 1, y - 1, OBLIQUE_VALUE);
// 右上
addNeighborNodeInOpen(mapInfo,current, x + 1, y - 1, OBLIQUE_VALUE);
// 右下
addNeighborNodeInOpen(mapInfo,current, x + 1, y + 1, OBLIQUE_VALUE);
// 左下
addNeighborNodeInOpen(mapInfo,current, x - 1, y + 1, OBLIQUE_VALUE);
}
/**
* 添加一个邻结点到open表
*/
private void addNeighborNodeInOpen(MapInfo mapInfo,Node current, int x, int y, int value)
{
if (canAddNodeToOpen(mapInfo,x, y))
{
Node end=mapInfo.end;
Coord coord = new Coord(x, y);
int G = current.G + value; // 计算邻结点的G值
Node child = findNodeInOpen(coord);
if (child == null)
{
int H=calcH(end.coord,coord); // 计算H值
if(isEndNode(end.coord,coord))
{
child=end;
child.parent=current;
child.G=G;
child.H=H;
}
else
{
child = new Node(coord, current, G, H);
}
openList.add(child);
}
else if (child.G > G)
{
child.G = G;
child.parent = current;
// 重新调整堆
openList.add(child);
}
}
}(7) 回溯法绘制路径
private void drawPath(int[][] maps, Node end)
{
if(end==null||maps==null) return;
System.out.println("总代价:" + end.G);
while (end != null)
{
Coord c = end.coord;
maps[c.y][c.x] = PATH;
end = end.parent;
}
}(8) 开始算法,循环移动结点寻找路径,设定循环结束条件,Open表为空或者最终结点在Close表
public void start(MapInfo mapInfo)
{
if(mapInfo==null) return;
// clean
openList.clear();
closeList.clear();
// 开始搜索
openList.add(mapInfo.start);
moveNodes(mapInfo);
}
/**
* 移动当前结点
*/
private void moveNodes(MapInfo mapInfo)
{
while (!openList.isEmpty())
{
Node current = openList.poll();
closeList.add(current);
addNeighborNodeInOpen(mapInfo,current);
if (isCoordInClose(mapInfo.end.coord))
{
drawPath(mapInfo.maps, mapInfo.end);
break;
}
}
}