Skip to content

3D Advecting Field Loop

Jump to bottom Edit New page
evazlimen edited this page Sep 23, 2023 · 1 revision

3D Advecting Field Loop

This test illustrates the ability of a code to preserve $B_{z}$ = 0 (and thus $\nabla \cdot B = 0$) for all time. Parameters from Gardiner & Stone 2008. The test consists of a magnetic loop of magnetic amplitude 0.001 and radius 0.3. The density and pressure are initally 1.0, and the velocity is $\hat{x}$ + $\hat{y}$ + 2 $\hat{z}$. This test is performed with the mhd build (cholla/builds/make.type.mhd). Full initial conditions can be found in cholla/src/grid/initial_conditions.cppunder Advecting_Field_Loop().

Parameter file: (cholla/examples/3D/advecting_field_loop.txt)

#
# Parameter File for an MHD Advecting Field Loop as defined in
# [Gardiner & Stone 2008](https://ui.adsabs.harvard.edu/abs/2008JCoPh.227.4123G/abstract)
#

################################################
# number of grid cells in the x dimension
nx=128
# number of grid cells in the y dimension
ny=128
# number of grid cells in the z dimension
nz=256
# final output time
tout=2.0
# time interval for output
outstep=2.0
# name of initial conditions
init=Advecting_Field_Loop
# domain properties
xmin=-0.5
ymin=-0.5
zmin=-1.0
xlen=1.0
ylen=1.0
zlen=2.0
# type of boundary conditions
xl_bcnd=1
xu_bcnd=1
yl_bcnd=1
yu_bcnd=1
zl_bcnd=1
zu_bcnd=1
# path to output directory
outdir=./

#################################################
# Parameters for advecting field loop problem
# initial density
rho=1.0
# velocity in the x direction
vx=1.0
# velocity in the y direction
vy=1.0
# velocity in the z direction
vz=2.0
# initial pressure
P=1.0
# amplitude of the loop/magnetic field background value
A=0.001
# Radius of the Loop
radius=0.3

# value of gamma
gamma=1.666666666666667

Upon completion, you should obtain 2 output files. The initial and final densities (in code units) of a slice along the y-midplane is shown below. Examples of how to plot projections and slices can be found in cholla/python_scripts/Projection_Slice_Tutorial.ipynb.
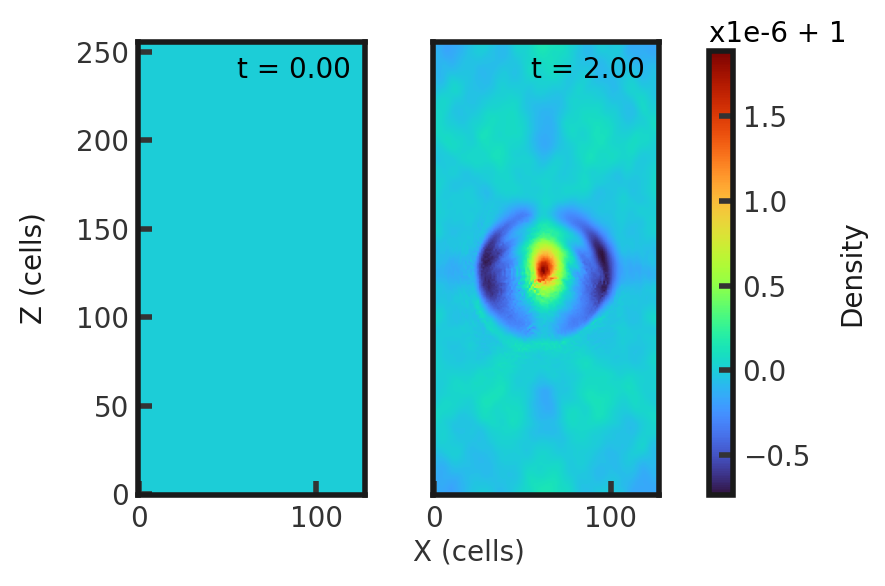
Two 2D histograms side by side, showing density of cells in the z direction vs cells in x direction. The leftmost is the initial density plot with a value of 1 for each cell. The rightmost plot is the final density plot at t = 0.2 with a circle of radius 15 cells of higher density (1.000002) in the center of the plot. This region is directly ringed by a region of lower density (0.999999) with a width of 30 cells. The remaining cells have a value of 1
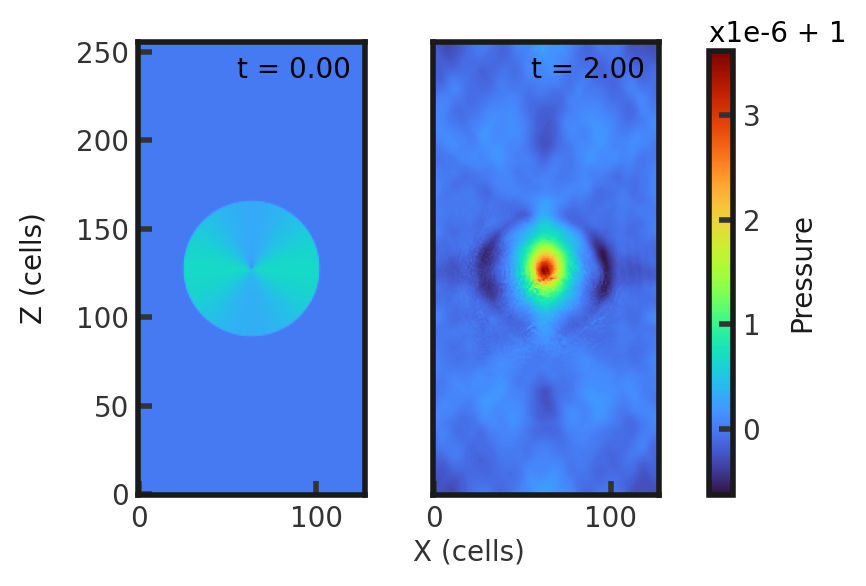
Two 2D histograms side by side, showing pressure of cells in the z direction vs cells in x direction. The leftmost is the initial pressure plot with circle of density 1.000001 and radius 40 centered on (64, 128). The circle varies slightly in density. The remaining cells have a density of 1. The rightmost plot is the final density plot at t = 0.2 with a circle of radius 15 cells of higher pressure (1.000004) in the center of the plot. There is a small gap before a ring of lower pressure (0.999999) of width 10. The remaining cells vary between 1.000000 and 1.000001 without any sharp transitions.

We can take a slice along the y-midplane of the z component of the magnetic field, averaging the value over the slice, at more frequent outputs to test how well the code preserves $B_{z}$ = 0:

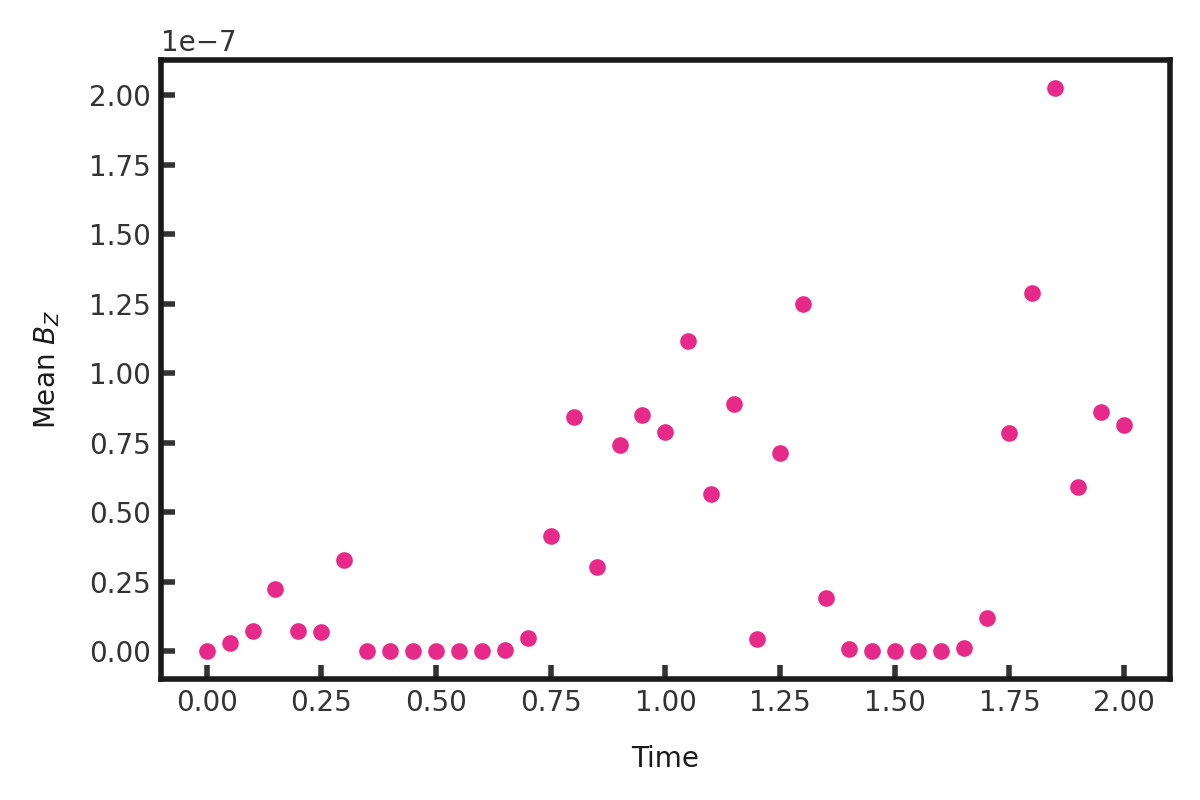A scatter plot of B_z vs time. B_z is on the order of 1e-7, gradually increasing with time. By changing the outstep to 0.05, you will obtain 41 output files and can obtain the below animation (10 fps):
advecting-field-loop-density.mp4

We see the loop moving to the upper right, prompting fluctuations in the background as it transitions out of view before returning in the lower left.

Add a custom footer

Cholla Wiki

Physics

Testing

Examples

Advanced

In progress

Contributing

Clone this wiki locally